Kedu ihe bụ ọkụ eletrik? Cheta nke mbụ, gịnị bụ nkọwa nke ugbu a anyị mụtara?

N’ụzọ dị mfe, mmegharị ntụzịaka nke ụmụ irighiri chajị n’ime onye nduzi bụ ọkụ eletrik.

Only when a substance has charged particles that can move freely, it can transmit electric current—that is, conduct electricity. These charged particles that participate in conduction are called carriers. For metals, for example, only the outer electrons of atoms can act as carriers.

The “directional movement” in the definition of electric current is often misunderstood. Many people think it refers to movement with a certain direction, of course not! Doesn’t the direction of movement of the electrons in the AC circuit change?

N’ezie, orienteering bụ ihe metụtara “random ije”!

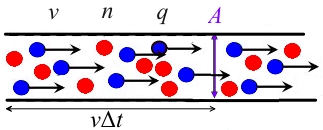
Since electrons are microscopic particles, they must be in thermal motion all the time. Thermal motion is a random motion, as shown in the figure below.

Ntugharị a na-adị ngwa ngwa n’ezie. Dị ka ihe atụ, na ọla na ụlọ okpomọkụ, ọsọ nke eletrọnịkị thermal ije bụ n’usoro nke narị narị kilomita kwa nkeji!

If you look closely at this random movement, you will find that the direction of movement of each particle is random at any moment. If you add up the velocity vectors of these particles, the result is almost zero.

Ugbu a tinye oghere eletrik na onye na-eduzi, na eletrọn na-ebuli mmegharị ntụziaka na ndabere nke mmegharị enweghị usoro. Na-eche na ọkụ eletrik dị n’aka ekpe ruo oge ụfọdụ, mmegharị nke electrons dị ka nke a. Bọọlụ na-acha uhie uhie na-anọchi anya atọm ọla na lattice kristal, na ntụpọ na-agba ọsọ na-anọchi anya eletrọn efu.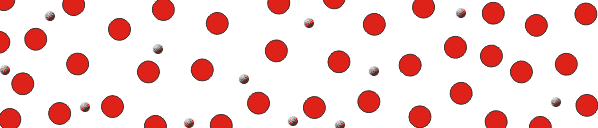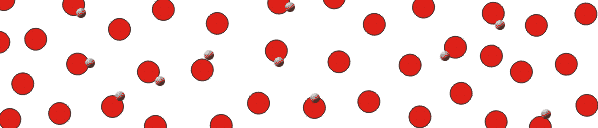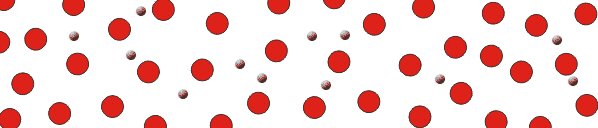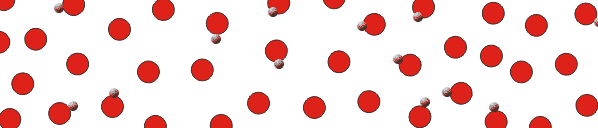

Ọ na-adị ngwa ngwa? Nke ahụ bụ n’ihi na mmegharị eletrọnịkị na-adị ngwa ngwa n’ezie! Ma n’ezie, mmegharị ahụ na-enweghị usoro, nke na-eme ka ọnụ ọgụgụ buru ibu nke ya, adịghị etinye aka na ugbu a. Mgbe a na-ewepụ mmegharị ahụ na-enweghị usoro, ndị ọzọ dị ka ngwa ngwa anya n’okpuru.

Indeed, the directional movement of electrons is much slower than the speed of thermal movement. This “grinding” movement of electrons is called drift, or “drift”. Sometimes, electrons will run in the opposite direction because of collisions with atoms. But in general, electrons move in one direction.

Ọ bụrụ na mpaghara ọkụ eletrik na-agbanwe ụzọ, ntụzịaka nke ịkpagharị eletrọn ga-agbanwekwa.

Ya mere, ụdị ntụgharị ntụzịaka a pụtara na nchikota ọsọ nke ndị electron niile na-ekere òkè na nduzi n’oge ụfọdụ abụghị efu, kama ọ bụ n’ozuzu ya n’akụkụ ụfọdụ. Enwere ike ịgbanwe ntụzịaka a n’oge ọ bụla, nke ahụ bụkwa ikpe nke alternating current.

Therefore, current is not so much the “directional movement” of electric charge as it is the “collective movement” of electric charge.

The magnitude of the current in the conductor is expressed by the current intensity. The current intensity is defined as the amount of electricity passing through the cross-section of the conductor in a unit time, namely

Anyị amụtala ụfọdụ ọnụọgụ anụ ahụ nwere okwu “ike” dị ka ike ọkụ eletrik na ike induction magnetik. Ha na-anọchi anya nkesa kwa nkeji oge, mpaghara otu (ma ọ bụ otu olu, otu akụkụ siri ike). Otú ọ dị, okwu ahụ bụ “ike” na ike dị ugbu a adịghị egosipụta òkè ugbu a nke mpaghara ahụ.

In fact, another physical quantity is responsible for the distribution of current to area, which is current density.

Since the essence of electric current is the directional movement of electric charge, there must be a certain relationship between current intensity and drift speed!

In order to obtain this relationship, we must first clarify a concept-carrier concentration, that is, the number of carriers in a unit volume, which is expressed by .

It is assumed that the conductor cross section is, the carrier concentration is, the drift velocity is, and the charged charge is.

Mgbe ahụ, ụgwọ dị na onye nduzi n’akụkụ aka ekpe nke elu bụ, na ụgwọ ndị a ga-agafe n’elu n’ime oge ụfọdụ, ya mere.

This is a microscopic expression of current intensity.

Current density is the apportionment of current to area, so the magnitude of current density is, but it is defined as a vector, and the direction is the direction of the drift velocity vector of the positively charged carriers, so the drift of electrons in the metal can be obtained from this Speed, as an example below.

Tụlee waya ọla kọpa, na-eche na atom ọla kọpa ọ bụla na-enye ihe elektrọn dị ka ihe na-ebu. E nwere 1 mol ọla kọpa, olu ya bụ, molar uka bụ, njupụta bụ, mgbe ahụ ihe na-ebu ịta nke ọla kọpa waya bụ.

Ebe Avogadro si na-adịgide adịgide. A na-achọta njupụta nke ọla kọpa, na uru a na-enweta site n’iji dochie ya bụ ihe dịka otu / cubic mita.

Assuming that the radius of the copper wire is 0.8mm, the current flowing is 15A, =1.6 C, and the drift velocity of electrons is calculated as

Enwere ike ịhụ na ọsọ nke eletrọn dị obere n’ezie.

For those who study circuits, the above is the complete definition of current.

But in physics, the above definition of current is actually only a narrow definition. More general currents are not limited to conductors, as long as the movement of electric charges is current. For example, when the electrons of a hydrogen atom move around the nucleus, an electric current is formed in its orbit.

Ka e were ya na ọnụ ọgụgụ nke ụgwọ eletrọnịkị bụ na oge mmegharị ahụ bụ. Mgbe ahụ oge ọ bụla nke na-agafe, a na-enwe nnukwu ego na-agafe na mpaghara ọ bụla nke akaghị aka, ya mere, ike ugbu a na-adabere na mmekọrịta dị n’etiti oge, ugboro na angular ọsọ, na ugbu a nwekwara ike ịkọwa dị ka

Maka ihe atụ ọzọ, diski metal ebubo, na-atụgharị gburugburu axis ya, na-etolitekwa akaghị mmiri na radii dị iche iche.

This kind of current is not a normal conduction current and cannot generate Joule heat! Can not form a real circuit.

Ma ọ bụghị ya, ị ga-enye m ngụkọ nke oke joule okpomọkụ na-emepụta kwa nkeji site na electrons nke atom hydrogen?

N’ezie, ihe dị ugbu a na oghere adịghị eju iwu Ohm. N’ihi na, maka ọkụ eletrik na-emepụta site na mmegharị nke ihe ndị a na-akwụ ụgwọ na oghere, ndị na-ebu ya anaghị ejikọta ya na lattice na metal, n’ihi ya, oghere enweghị nguzogide na ọ dịghị conductance.

Ntugharị nke ụgwọ eletrik na-emepụta ọkụ eletrik, na ọkụ eletrik n’onwe ya na-akpali ọkụ eletrik. Nke a dị mfe ịkpata nghọtahie. Ya mere, ọtụtụ ndị mmadụ na-eche na a ga-ekpughere oghere eletrik nke ụmụ irighiri ihe ndị na-emepụta ọkụ eletrik. Ma n’ezie, n’ihi na conduction ugbu a na a n’ozuzu ndu, na-ebu na-eru na a ndabere mejupụtara ọnụ ọgụgụ dị ukwuu nke mma ebubo metal ion, na onye nduzi n’onwe ya na-anọpụ iche!

Anyị na-akpọkarị ụdị ugbu a pụrụ iche “ụgbụ a dakọtara”. Ihe ha nhata ebe a pụtara na ọ na-ewepụta oghere ndọta n’otu aka ahụ dị ka ihe na-eme ugbu a na-ebugharị nkịtị!

Reminder: Do not confuse the “equivalent current” here with the “equivalent circuit” in circuit analysis

N’ezie, mgbe mbụ anyị mụrụ ihe ndọta, ọkụ eletrik dị na iwu Biot-Saffar bụ ọkụ eletrik a na-achịkọta ọnụ nke nwere ugbu a. N’ezie, nhazi nke ugbu a na nha nha Maxwell na-ezokwa aka na mkpokọta ugbu a.

Those who have studied the photoelectric effect know that when the photoelectron drifts from the cathode to the anode, if the influence of air is ignored, this current is caused by the movement of electric charges in the vacuum, and there is no resistance, so it is not restricted by Ohm’s law.

Yabụ, nke a ọ bụ naanị ihe gbasara ọkụ eletrik na physics?

Mba! E nwekwara ụdị abụọ, ya bụ magnetizing current na displacement current.

They are also two equivalent currents, which, as the name suggests, are also introduced to explain magnetism. In other words, they have broken away from the basic characteristic of the current “charge movement”!

That’s amazing! There is no electric charge movement, so why can it be called an electric current?

Don’t worry, and listen to me slowly.

Let’s look at the magnetizing current first.

It was found that magnetism is caused by the movement of electricity (not considering the explanation of magnetism by the intrinsic properties of spin for the time being). In order to explain natural magnetism, French physicist Ampere put forward the hypothesis of “molecular circulation”.

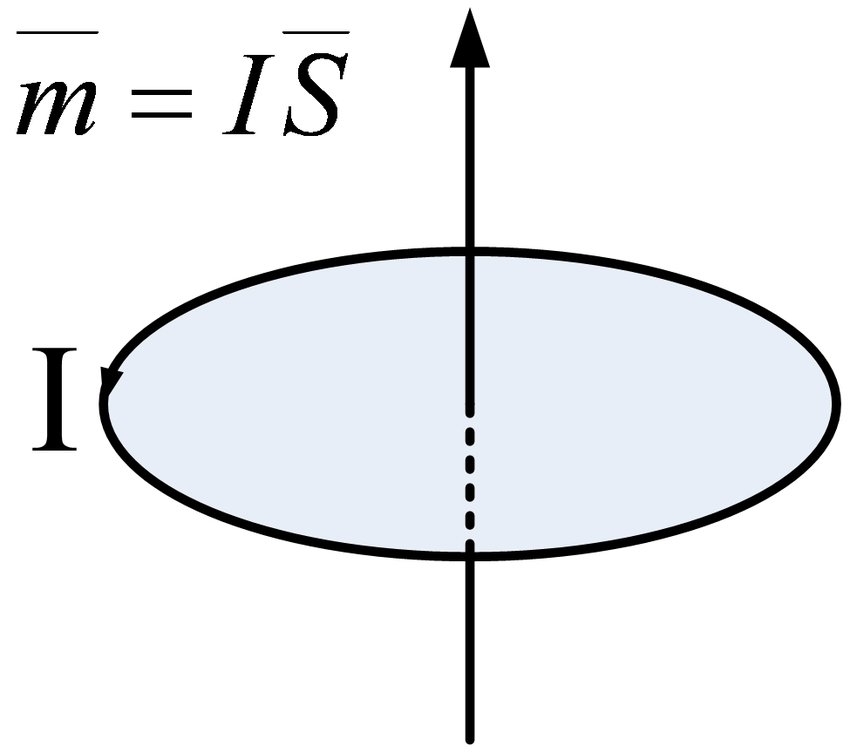
As shown in the figure below, any atom or molecule can be regarded as having an electric charge rotating around the center, forming a tiny loop current, that is, “molecular circulation”.

Dị ka iwu na ọkụ eletrik na-akpali oghere magnetik, nkesa molekụla a ga-emepụta ọnụọgụ anụ ahụ nke a na-akpọ oge magnetik. Ogo ya bụ mpaghara kpuchiri ya site na mgbasa ozi molekụla na-abawanye site na nha nke ugbu a nke mgbasa ozi molecular, na ntụzịaka ya dị na mmekọrịta okirikiri nke aka nri na ntụzịaka nke mgbasa, ya bụ.

Obviously, the direction of the magnetic moment is exactly along the direction of the magnetic field formed by the circulating current

.

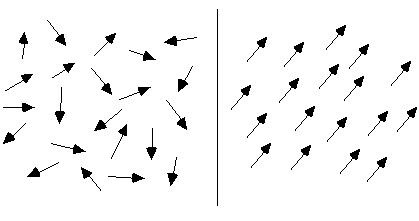
Under normal circumstances, the arrangement of the molecular circulation of a substance is chaotic, so the substance is not magnetic, as shown on the left side of the figure below. When subjected to an external magnetic field, these molecular circulations will be approximately neatly arranged. As shown on the right side of the figure below, their magnetic moments are arranged in one direction as much as possible, just like countless small magnetic needles gathered together to form a total magnetic field, and the whole material composed of them becomes magnetic.

Ka e were ya na e nwere magnetik cylindrical, a na-ahazi mgbasa nke mkpụrụ ndụ dị n’ime nke ọma, na akụkụ nke mgbasa ozi nke ọ bụla na nsọtụ akụkụ magnet na-ejikọta ọnụ iji mepụta nnukwu mgbasa ozi, dị ka e gosiri na foto dị n’okpuru ebe a.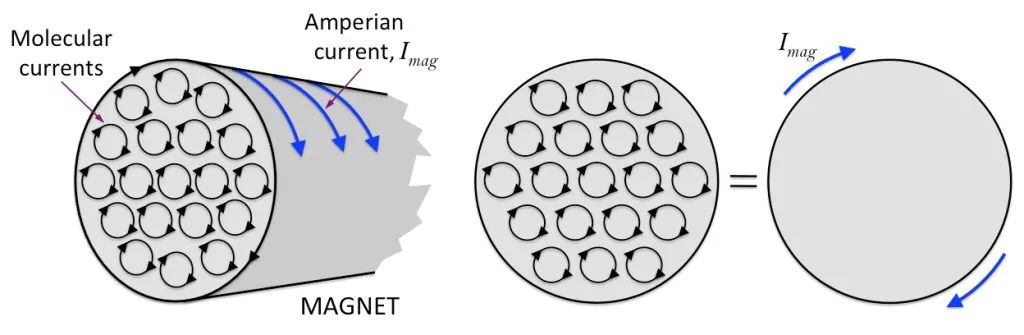

Based on this, we can think that a bar magnet is like an energized solenoid. In other words, there is an invisible current entangled on the surface of the magnet! This kind of current cannot be connected and used. It is confined to the surface of the magnet. We call it “binding current” or “magnetizing current”.

Ya mere, magnetizing ugbu a bụ ihe dị ugbu a, n’ihi na ọ bụ otu ihe ahụ na-emepụta site na mmegharị nke ezigbo ụgwọ eletrik, nke nwere ike ịmepụta oghere magnet!

Ka anyị leba anya na mgbapụ ugbu a ọzọ.

Dị ka Ampere’s loop theorem si kwuo, ihe jikọrọ ike ndọta na ụzọ mechiri emechi bụ nha nha nke njupụta dị ugbu a n’elu elu ọ bụla gbara agba nke ụzọ a, ya bụ, a na-akpọ theorem a Stokes’ theorem na mgbakọ na mwepụ. Ọ na-agwa anyị na akụkụ nke vector n’akụkụ ụzọ ọ bụla mechiri emechi ga-abụrịrị nhata na ntugharị nke curl ya (ebe a) n’elu ala ọ bụla ejiri ụzọ mechiri emechi.

Ebe ọ bụ usoro mgbakọ na mwepụ, ọ ga-abụrịrị eziokwu mgbe niile, n’ihi na mgbakọ na mwepụ bụ usoro ezi uche dabere na axioms.

Therefore, the Ampere Loop Theorem must always hold!

Otú ọ dị, ọkà mmụta sayensị Scotland bụ Maxwell chọpụtara na mgbe ọ na-eche sekit na-adịghị agbanwe agbanwe ihu, Ampere loop theorem na-emegiderịta onwe ya.

Ihe na-emekarị ugbu a na-akwụghị ụgwọ na-apụta n’oge ịchaji na nchaji nke capacitor. Dị ka egosiri na foto dị n’okpuru ebe a, enwere ọkụ na-adịghị akwụ ụgwọ n’oge obere oge nke chaja capacitor.

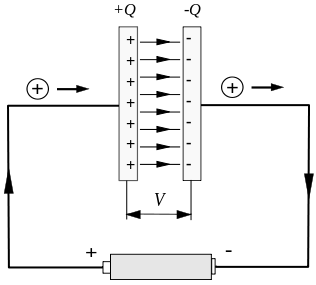
Ma sekit ahụ kwụsịrị n’etiti efere capacitor, nke ga-eme ka nsogbu dị njọ.

Suppose we consider a closed path that bypasses the wire, as shown in the figure below, the circle marked by C, and the curved surface with it as the boundary can be arbitrarily selected. In the figure, the circular plane enclosed by C itself and across the capacitor are selected. The curved surface of the left plate.

Dị ka okirikiri okirikiri dị n’elu, enwere ike ịhụ na dị ka akụkụ ahụ gbagọrọ agbagọ, ma dị ka akaghị aka nke ike magnetik, uru ya kwesịrị ikpebi!

Olee otu esi eme?

Maxwell believes that the Ampere’s loop theorem must be established. Now that there is a problem, it must be because a part of the current has not been discovered by us before, but it does exist!

So, how to find out this part of the current?

Since the problem is between the plates, start from between the plates.

Through analysis, Maxwell found that regardless of charging or discharging, there is a physical quantity between the capacitor plates at all times that is synchronized with the magnitude and direction of the current. It is the time derivative of the flux of the electric displacement vector, that is, it is defined as the displacement current.

If it is considered that this part is the part of the current that has not been discovered before, then the complete current is now. That is to say, although the circuit between the plates is disconnected, the derivative of the electric displacement flux and the sum of the current together, as a whole , Ensure the continuity of the current at all times.

N’ịlaghachi na esemokwu gara aga, anyị maara ugbu a na, dị ka ihe ndị Stokes’ theorem chọrọ si dị, mgbe ị na-agbakọ njupụta nke njupụta dị ugbu a maka elu mechiri emechi, a ga-atụlekwa njupụta nke mgbapụ ugbu a, ya bụ, ampere loop zuru ezu. Theorem bụ ya mere, Site na “ịchọpụta” ihe ọhụrụ a dị ugbu a, a na-edozi nsogbu nke Ampere Loop Theorem!

The reason why “introduction” is not used here, but “discovery” is used here. What I want to emphasize is that this kind of current is not a mathematical compensation, but a real thing, but it has not been discovered before.

Gịnị mere o ji dịrị na mbụ? N’ihi na ọ na-arụ ọrụ dị ka ọkụ eletrik, dị ka a conduction current, ọ na-akpalite magnetik n’otu aka ahụ, ma e wezụga na ọ dịghị mmegharị nke ụgwọ eletrik, ọ dịghị waya achọrọ, ọ dịghịkwa okpomọkụ Joule nwere ike ịmepụta, ya mere a na-eleghara ya anya!

Mana ọ dị n’onwe ya, debe profaịlụ dị ala, ọ na-ama jijiji na oghere ndọta mgbe niile!

N’ikwu ya n’ụzọ ọzọ, mgbe anyị na-eche ihu magnetik ihu, nkọwa mbụ nke ugbu a dị warara. Ihe kachasị mkpa nke ọkụ eletrik abụghị mmegharị nke ụgwọ eletrik, ọ kwesịrị ịbụ ihe nwere ike ime ka oghere magnetik mee ihe.

So far, the several forms of current have been introduced. They all exist objectively, and what they have in common is that all currents can equally excite the magnetic field.